Solution for 2xy=32 equation Simplifying 2x y = 32 Solving 2x y = 32 Solving for variable 'x' Move all terms containing x to the left, all other terms to the rightGraph 2x4y=32 Solve for Tap for more steps Subtract from both sides of the equation Divide each term by and simplify Tap for more steps Divide each term in by The slope of the line is the value of , and the yintercept is the value of Slope yintercept Slope yinterceptSolution for 2y=32 equation Simplifying 2y = 32 Solving 2y = 32 Solving for variable 'y' Move all terms containing y to the left, all other terms to the right Divide each side by '2' y = 16 Simplifying y
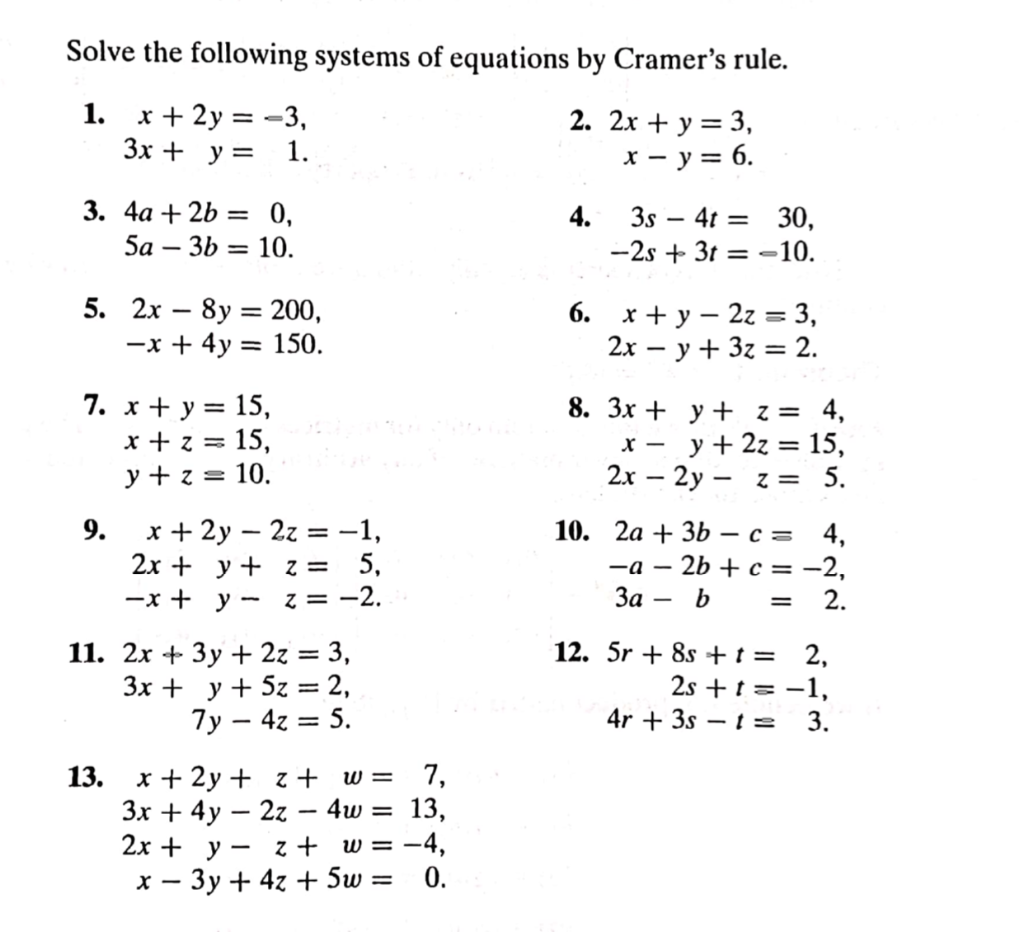
Solve The Following Systems Of Equations By Cramer S Chegg Com
32(x+y)2-2x-2y
32(x+y)2-2x-2y-Equations Tiger Algebra gives you not only the answers, but also the complete step by step method for solving your equations (32x^2y^312xy^28xy)/4xy so that you understand better Let $x, y \in \mathbb R$ s t $x^2y^2=2x2y2$ Find the largest possible value of $x^2y^2\sqrt{32}$ I know this is a duplicate of another question, but that




Solved 4x 2 Y 62 6x 3y 3z 37 3x Y Z 1 5x 4y 2z 5 2x Y Z 2 34 2x 4y Z 10 X 2y Z 1 X 3y 2z 0 1 12x Q
The equation is To change the expressions (x 2 2x) and (y 2 4y) into a perfect square trinomial,add (half the x coefficient)² and add (half the y coefficient)²to each side of the equation Compare the standardform of ellipse a 2 > b 2 If the larger denominator is under the "y " term, then the ellipse is verticalCenter (h, k )a = length of semimajor axis, b = length of semi x=4 or x=4 First divide throughout by 2 to get x^2=16 Now take the square root on both sides to get x=4 You may now substitute the values of x=4 and x=4 back into the original equation and check that it satisfies the equation which it does3x2y=2;2xy=8 Simple and best practice solution for 3x2y=2;2xy=8 Check how easy it is, to solve this system of equations and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history2^(2x)12*2^(x)32=0 That is a quadratic with variable = 2^x(2^x)^2 12(2^x) 32 = 0Let w = 2^xPlot 3x^22xyy^2=1 WolframAlpha Assuming "plot" is a plotting function Use as referring to geometry instead
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreNow, y = x± q x2 −4(x2 −7) 2 = x± √ 28−3x2 2 Take the positive root since y(1) = 3 The restriction on x would be that 28−3x2 ≥ 0 Therefore, − s 28 3 < x < s 28 3 5 Problem 15 (xy2 bx2y)dx(xy)x2 dy = 0 First, for this to be exactY 2 ((((32•(x 9))•——)•x)•y)2 2 Step 2 Equation at the end of step 2 y 2 (((2 5 x 9 • ——) • x) • y) 2 2 Step 3 Dividing exponents 31 2 5 divided by 2 1 = 2 (5 1) = 2 4 Equation at the end of step 3 ((16x 9 y 2 • x) • y) 2 Step 4 Step 5 Pulling out like terms



2



Www Kingphilip Org Site Handlers Filedownload Ashx Moduleinstanceid 1434 Dataid 60 Filename Lesson 7 3 practice a Pdf
Click here 👆 to get an answer to your question ️ 32(xy)22x2y factorise bebydevi58 bebydevi58 3 weeks ago Math Secondary School answered 32(xy)22x2y factorise 1 See answer bebydevi58 is waiting for your help Add your answer and earn points I will assume that you want d/(dx)(x^2y^2) By implicit differentiation d/(dx)(x^2y^2)=2xy^22x^2y(dy)/(dx) We are assume that y is some function or functions of x Think of it as x^2y^2=x^2*("some function")^2 To differentiate this, you'd need the product rule and the chain rule d/(dx)x^2*("some function")^2=2x*("some function")^2x^2*2("someGuest 0 users composing answers



Solved Solve Begin Array L W X Y Z 2 W



Secure Media Collegeboard Org Digitalservices Pdf Ap Apcentral Ap15 Calculus Ab Q6 Pdf
If your question is "What is x in the equation", then x = 16 2x = 32 8 8 2x = 32 x = 32/2 = 16 EDIT I would like to remind you about PEMDAS rule to follow before making such calculations If you think you would feel satisfied by using a calcuGraph y=x^212x32 y = x2 12x 32 y = x 2 12 x 32 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for x 2 12 x 32 x 2 12 x 32 Tap for more steps Use the form a x 2 b x c a x 2 b x c, to find the values of a a, b b, and c cStep by step solution of a set of 2, 3 or 4 Linear Equations using the Substitution Method y=2x1;2x2y=22 Tiger Algebra Solver
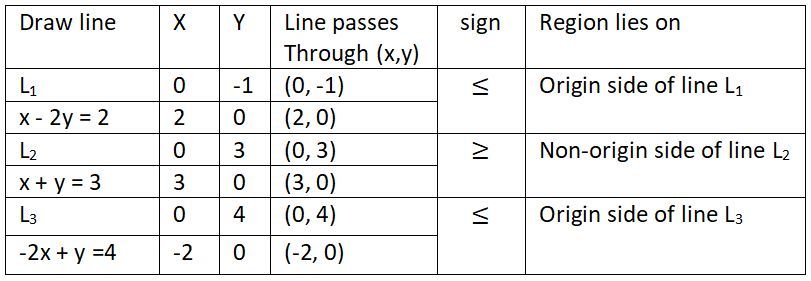



Solve The Following System Of Inequations Graphically X 2y Le 2 X Y Ge 3 2x Y Le 4 X Ge 0 Y Ge 0 Snapsolve



3
Thanks for contributing an answer to Mathematics Stack Exchange!2x4y=32;6x2y=12 Simple and best practice solution for 2x4y=32;6x2y=12 Check how easy it is, to solve this system of equations and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homeworkFind the Vertex y=x^212x32 Rewrite the equation in vertex form Tap for more steps Complete the square for Tap for more steps Use the form , to find the values of , , and Consider the vertex form of a parabola Substitute the values of and into the formula Simplify the right side




Schaum S Outline College Algebra Pdf Txt




9 Az Mor
This is easy if you don't insist on using Lagrange multipliers The normal to the ellipse at the point (x,y,z) is \nabla(x^2y^24z^2) = (2x, 2y, 8z) At minimum or maximum distance to the plane,Related » Graph » Number Line » Examples » Our online expert tutors can answer this problem Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Systemofequationscalculator 4x3y=1, 2x3y=2 en Related Symbolab blog posts Middle School Math Solutions – Simultaneous Equations Calculator Solving simultaneous equations is one small algebra step further on from simple equations Symbolab math solutions




If 2x 2 2y 2 2x 2y 1 0 Then What Is The Value Of X Y Quora




Solved 4x 2 Y 62 6x 3y 3z 37 3x Y Z 1 5x 4y 2z 5 2x Y Z 2 34 2x 4y Z 10 X 2y Z 1 X 3y 2z 0 1 12x Q
The Questions and Answers of If 22xy= 32 and 2x y= 16 then x2 y2a)9b)10c)11d)13Correct answer is option 'B' Can you explain this answer?Trigonometry Graph 2x^22y^2=32 2x2 2y2 = 32 2 x 2 2 y 2 = 32 Divide both sides of the equation by 2 2 x2 y2 = 16 x 2 y 2 = 16 This is the form of a circle Use this form to determine the center and radius of the circle (x−h)2 (y−k)2 = r2 ( x h) 2 ( y k) 2 = r 2 Match the values in this circle to those of the standardSolve Quadratic Equation by Completing The Square 52 Solving x210x16 = 0 by Completing The Square Subtract 16 from both side of the equation x210x = 16 Now the clever bit Take the coefficient of x , which is 10 , divide by two, giving 5 , and finally square it giving 25 Add 25 to both sides of the equation On the right hand side




Rd Sharma Solutions For Class 8 Chapter 7 Factorization Download Free Pdf



Http Ougouag Com 210 4 7 Pdf
Multiply x 2 y 2 y 2 − 2 x y x 2 times − x y − 2 x by multiplying numerator times numerator and denominator times denominator Cancel out x in both numerator and denominator Cancel out x in both numerator and denominator Factor the expressions that are not already factored2 x 2 − 2 x y 2 y 2 = ( x − y) 2 x 2 y 2 Again, we have a sum of squares on the right, and this can be 0 only if x, y (and therefore x − y) are all 0 Much more mechanically, we can use the Quadratic Formula For any fixed y, the solutions of x 2 − x y y 2 = 0 are x = y ± − 3 y 2 2Start your free trial In partnership with




Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy




Solutions To Maximum Minimum Problems
Let C 1 and C 2 be the centres of the circles x 2 y 2 – 2x – 2y – 2 = 0 and x 2 y 2 – 6x – 6y 14 = 0 respectively If P and Q are the points of intersection of these circles, then the area (in sq units) of the quadrilateral PC 1 QC 2 is (1) 9 (2) 8 (3) 4 (4) 6But avoid Asking for help, clarification, or responding to other answers =color(red)(4 x^4 y^6 (2^1x^2y^3)^color(red)(2 Here color(red)(2 needs to be multiplied with each term within the bracket =2^(1 color(red)(2))x^(2 color(red)(2)) y^(3 color(red)(2) =2^2 x^4 y^6 =color(red)(4 x^4 y^6



Http Www Pitt Edu Gdhart Algprobspoly2 Pdf



Q Tbn And9gcqwyaa3t Pdfs Okiqmu3brnemnyjkhlkijd2uylfxyqhlxgmdn Usqp Cau
51 Find the Vertex of y = x 2 4x32 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting "y" because the coefficient of the first term, 1 , is positive (greater than zero) Each parabola has a vertical line of symmetry 32x^3y^4 48x^2y Take out the GCF's first GCF 32 , 48 = 16 GCF x^3 , x ^2 = x^2 GCF y^4 , y = y so we have 16x^2y 2xy^3 3You can put this solution on YOUR website!
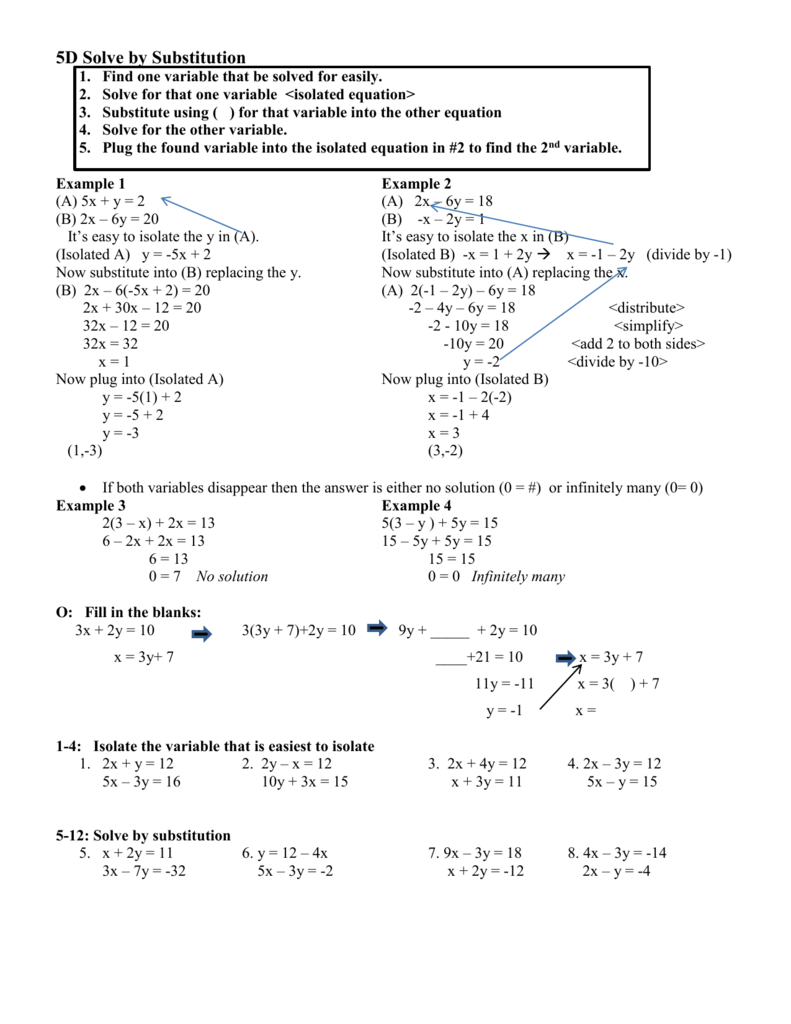



5d Solve By Substitution




Solve The Following Systems Of Equations By Cramer S Chegg Com
When I set y ( x) = A x u ( x) = A u x , (I think this is how you solve second order with variable coefficients) I got to the point u ″ ( x − x 3) 2 u ′ ( 1 − 2 x 2) = u ″ ( x − x 3) 2 u ′ ( 1 − 3 x 2) 2 u ′ x 2 = d d x u ′ ( x − x 3) 2 u ′ ( 1 − x 2) = 0 Then I let u ′ = w and got w ′ ( x − x 3 xy = 32 x=24, y= 8 When x>0, y Solve $$ (y^2 2x^2y)dx (2x^3 xy)dy = 0 $$ I tried to solve the differential equation in the following manner, but I am unable to arrive at




Differentiating Implicitly Use Implicit Chegg Com




Engineering Mathematics Notes
Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!1st x2y=0 2nd y=2x3Rewrite 2nd as follows 3rd 2xy=3 Multiply 1st by 2 to get 4th 2x4y=0 Subtract 4th from 3rd to get 5th 3y=3 y=1 Substitute this value into 1st to solve for x x2(1)=0 x=2 Solution (2,1) Cheers, Stan HSystemofequationscalculator 2x4y=2, 2xy =4 en Related Symbolab blog posts High School Math Solutions – Systems of Equations Calculator, Nonlinear In a previous post, we learned about how to solve a system of linear equations In this post, we will learn



Http Www Prosper Isd Net Cms Lib5 Tx Centricity Domain 52 4 3 elimination practice Pdf



2
Graph y^2=32x y2 = 32x y 2 = 32 x Rewrite the equation as 32x = y2 32 x = y 2 32x = y2 32 x = y 2 Divide each term by 32 32 and simplify Tap for more steps Divide each term in 32 x = y 2 32 x = y 2 by 32 32 32 x 32 = y 2 32 32 x 32 = y 2 32 Cancel the common factor of 32 32Get an answer for 'Solve 2^2x 12*2^x 32 = 0' and find homework help for other Math questions at eNotesCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history



What Is The Center Of The Ellipse Math Frac X Y 2 2 9 Frac X Y 2 16 1 Math Quora




15 Three Lines X 2y 3 0 X 2y 70 And 2x Y 4 0 Form The T Scholr
if 2^2xy=16 and 2^xy=32 find x * y answer is 6 New questions in Math Find Hcf of the 42 and 84 by listing factors uivopgmkgi come for F u n talk 18TTTT1 COS — i sin914 Show that9T1 cos —isin99=1T फ्रॉम द स्मालेस्ट टू डिजिट नंबर यूजिंग 2x Explanation 2x 6y = 32 5x −2y = 12 In order to use substitution, rearrange one of the equations 2x 6y = 32 2x = −6y 32 (Subtracted 6y from both sides) x = − 3y 16 (Divided both sides by 2) Now that we rearrange the equation, What is the center and radius of 2x^22y^2=32?



Http Www Pitt Edu Gdhart Alglectpoly2 Pdf
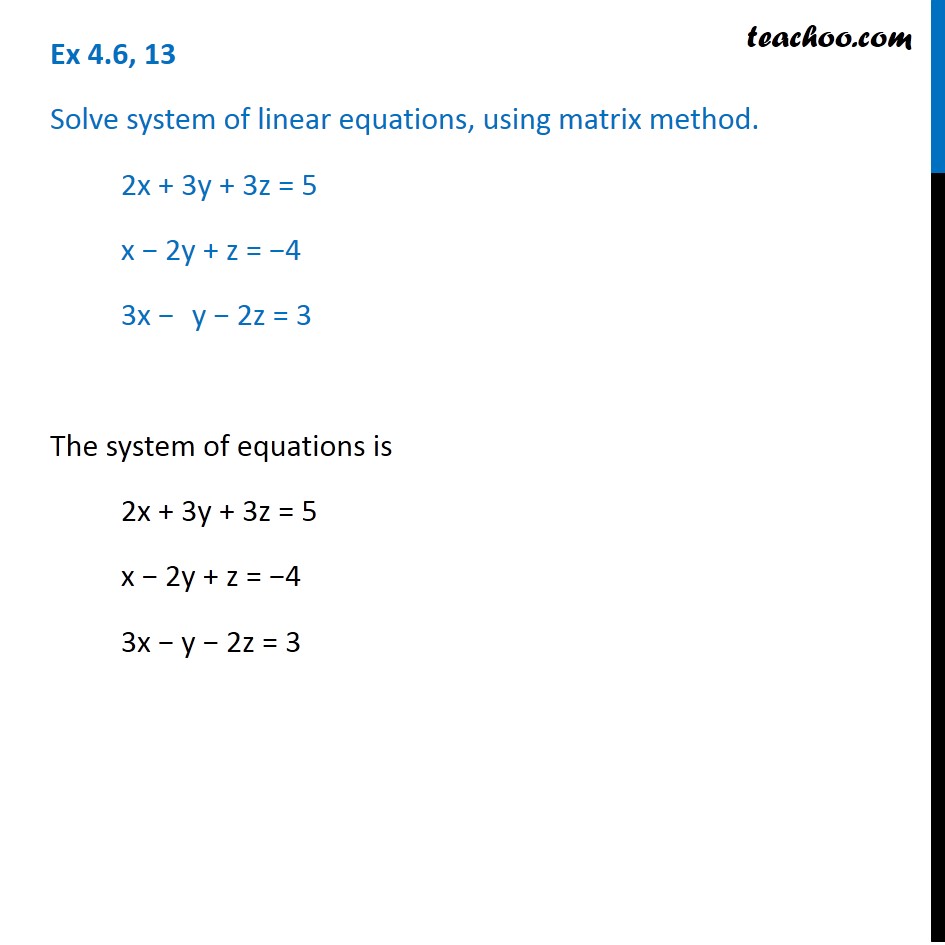



Ex 4 6 13 Solve Linear Equations Using Matrix Method Ex 4 6
The quadratic formula gives two solutions, one when ± is addition and one when it is subtraction x^ {2}2yxy^ {2}=0 x 2 2 y x y 2 = 0 This equation is in standard form ax^ {2}bxc=0 Substitute 1 for a, 2y for b, and y^ {2} for c in the quadratic formula,Are solved by group of students and teacher of Class 9, which is also the largest student community of Class 9Please be sure to answer the questionProvide details and share your research!




For X Y Belongs To N If 32x Y 1 3y 2x 1 Maths Inverse Trigonometric Functions Meritnation Com
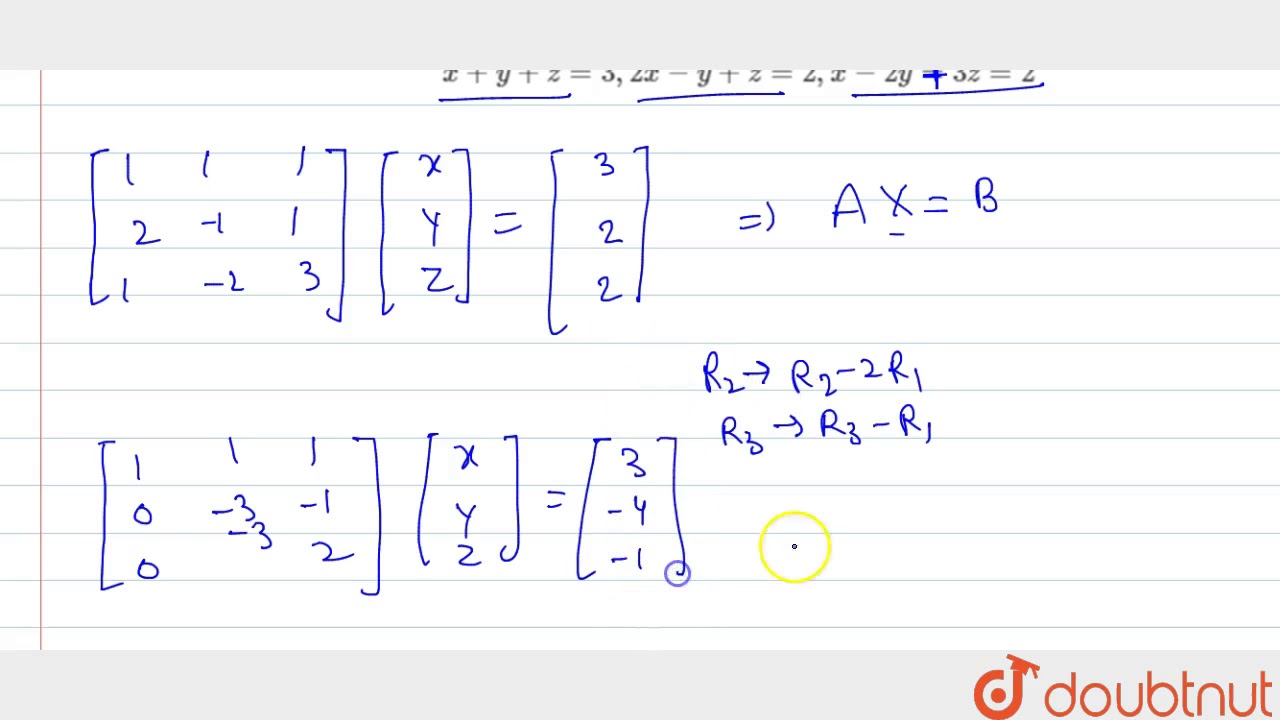



Solve The Following System Of Linear Equations By Matrix Method X Y Z 3 2x Y Z 2 X 2y 3z 2 Youtube
X 2 ( − 2 y − 8) x y 2 − 8 y = 0 This equation is in standard form ax^ {2}bxc=0 Substitute 1 for a, 2y8 for b, and y\left (8y\right) for c in the quadratic formula, \frac {b±\sqrt {b^ {2}4ac}} {2a} This equation is in standard form a x 2 b x c = 0




Solve Division Of Polynomials Step By Step Math Problem Solver




Solve Y 2 2x 2y Dx 2x 3 Xy Dy 0 Mathematics Stack Exchange
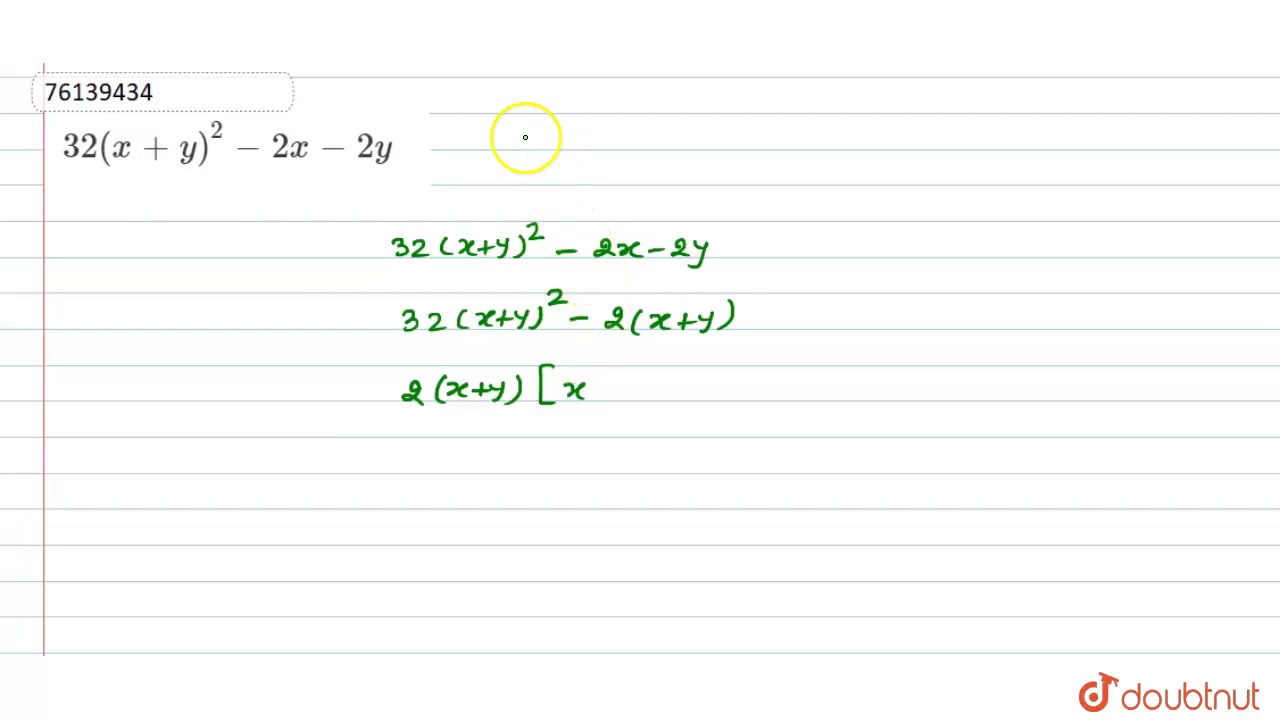



32 X Y 2 2x 2y Youtube




Vector Analysis By Alimkanwalimtinaa Issuu




Pdf Double Integrals Sheet A R F Academia Edu




Answered To Vs 2xy Xzy Xy 2 V Cy4cz 2 Bartleby




Beginarrayr 2x Y 3z 3 2x Y Z 5 X 2y 2z 22x 2z Gauthmath




Graph The Following Systems O See How To Solve It At Qanda




Find The Minimum Value Of X 2 4xy 4y 2 2z 2 Given That Xyz 32 Mathematics Stack Exchange



Http Users Math Msu Edu Users Holmesir M2552 Exam1 Reviewproblems Pdf




Sol Cap4 Baldor




Algebra Factorising Linear And Quadratics Ppt Download
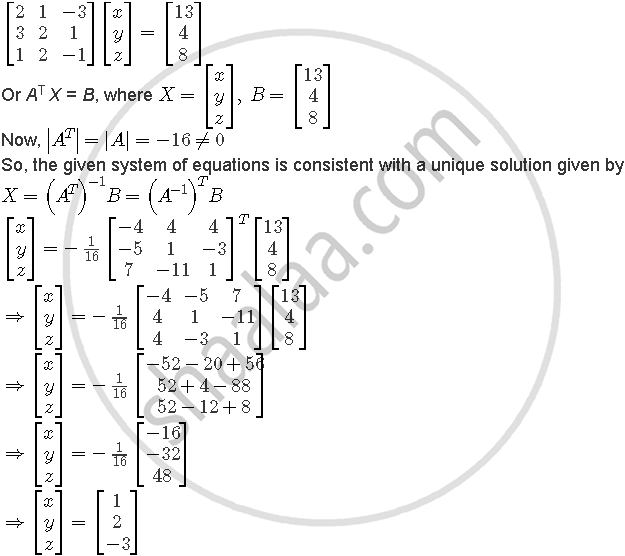



If A 2 3 1 1 2 2 3 1 1 Find A 1 And Hence Solve The System Of Equations 2x Y 3z 13 3x 2y Z 4 X 2y Z 8 Mathematics Shaalaa Com
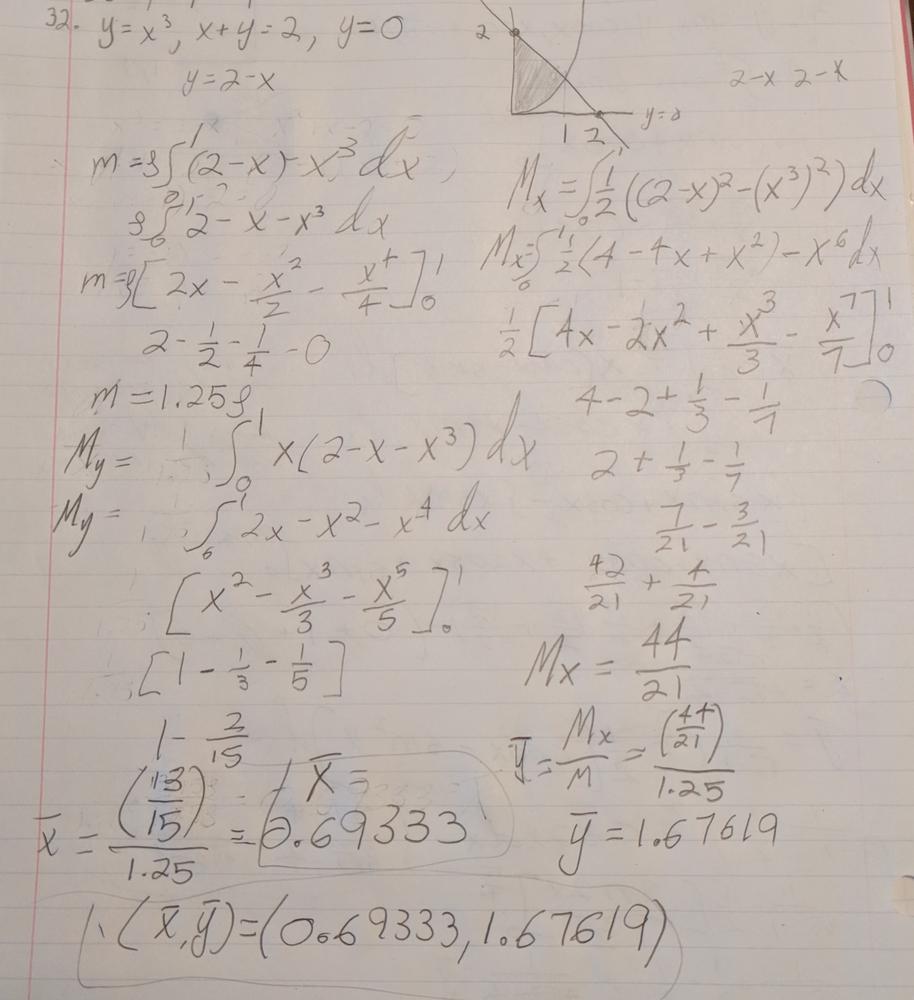



Find The Centroid Of The Region Bounded By The Given Curves Y X 3 X Y 2 Y 0 Homework Help And Answers Slader




2 2x Y 3z 2 X Y 2 3 3x 2y 3z 1 Gauthmath



Solved Find The Slope Of The Surface Z 2x Y X 2y At The Point 4 2 3 4 In Both The X Direction And The Y For F X Y Sin 2x 3 Course Hero



If Math X Y 2 Text And X 2 Y 2 2 Math What Is The Value Of Math Xy Math Could You Break It Down For Me Quora




Factorise The Following 32 X Y 2 2x 2y Brainly In



2




If 2x Y 2 5 3x Y 1 3 2 X 2y 1



Q Tbn And9gcqpt2b1vv70to Lfdbr3ccujmyvr Vdkumxv5jjwr7ln1ajiae7 Usqp Cau
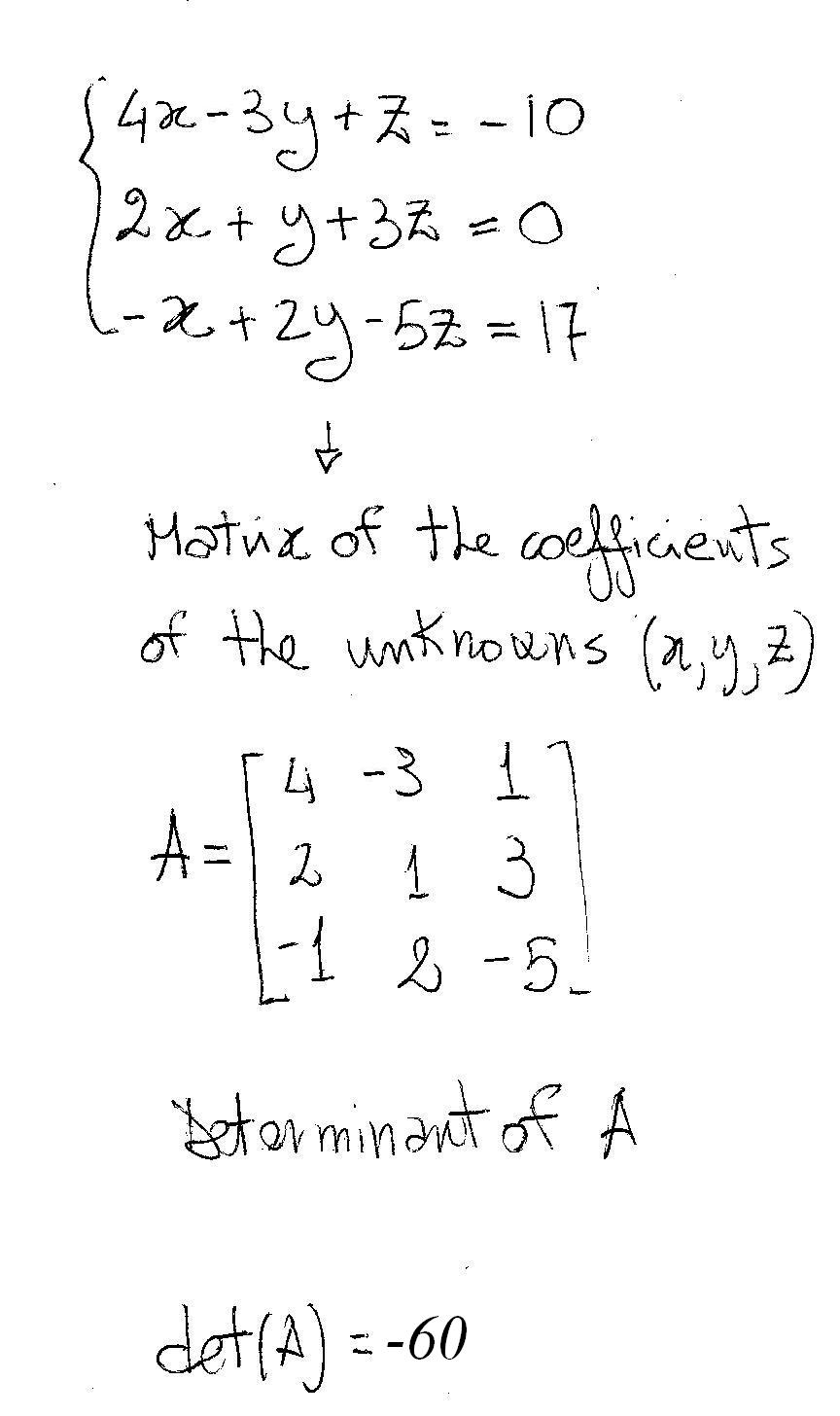



Solving A System Of Equations Using A Matrix Precalculus Socratic



Solve The System Of Linear Equations By Matrix Method X Y Z 3 2x Y Z 2 X 2y 3z 2 Mathematics Topperlearning Com Dqx3252pp




32 X Y 2 2x 2y




How To Solve Cyclic Differential Equations




Find The Equation Of Straight Line Which Passes Through The Intersection Of The Straight Lines 3x 2y 4 0 And X Y 2 0 And Forms A Triangle With The Axes Of Area 8 Sq Units Snapsolve




Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook



Mryangteacher Weebly Com Uploads 7 7 0 2 3 7 Optimization Notes And Hw Pdf



Pmt Physicsandmathstutor Com Download Maths A Level C4 Topic Qs Edexcel Set 1 C4 differentiation implicit differentiation Pdf
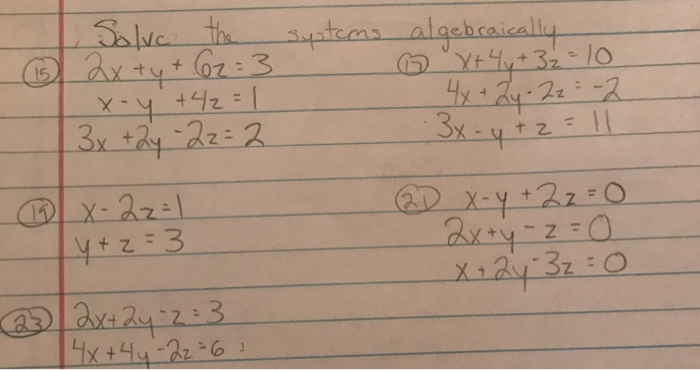



Solve The Systems 2x Y 62 3 1 X Y 42 1 3x 2y Chegg Com




Rd Sharma Solutions For Class 8 Chapter 7 Factorization Download Free Pdf




3x 2y 3 2x Y 16 Find The Values Of Determinants In The Given Equations D Dx Lisil 38 D 2 L Le Values According To Cramer S Rule 35 42 X X Y 5 7 6 Is The Solution 15



Www Tau Ac Il Levant Ode Solution 6 Pdf
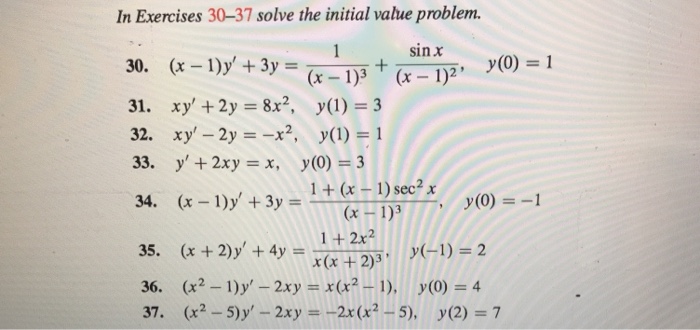



In Exercises 30 37 Solve The Initial Value Problem Chegg Com



Tinkutara Equation Editor Math Forum Question 4430




2 Using A 2r Y Z 4 3x 2y 32 16 1 X




Ns 2 6 Solve Each Sys Of E R X 2y 2 4 2x Y 32 8 2x Chegg Com




On Students Understanding Of Implicit Differentiation Based On Apos Theory Springerlink



Secure Media Collegeboard Org Digitalservices Pdf Ap Apcentral Ap15 Calculus Ab Q6 Pdf




Personal Lnde Page 32 33 Created With Publitas Com
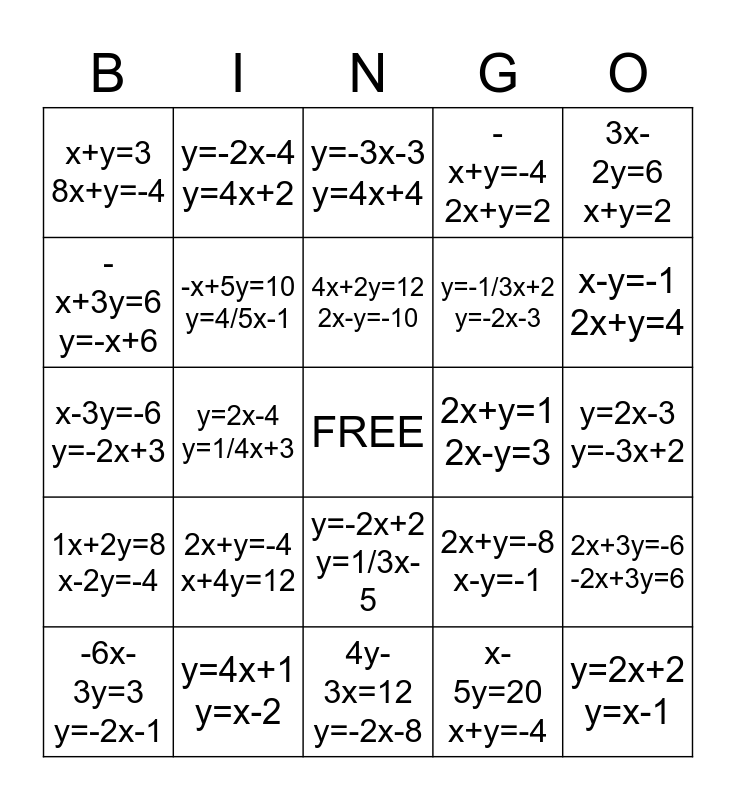



Solving Systems Of Equations By Graphing Bingo Card



Using Matrix Method Solve The System Of Equations 3x 2y 2z 3 X 2y 3z 6 2x Y Z 2 Studyrankersonline




Solutions To Implicit Differentiation Problems




32 X 2 Y 1 1 And 8 Y 16 4 X 2 0 Brainly In




University Calculus Elements With Early Transcendentals 1st Edition H



Find The Asymptotes Of The Following Curves Y 3 X 2y 2x Y 2 2x 3 7xy 3y 2 2x 2 2x 2y 1 0 Youtube




Let The Polar Coordinates Of The Point X Y Be R Math Theta Math Determine The Polar Coordinates For The Points A X Y B 2x 2y And C 3x 3y Homework Help



How Do You Solve 2x 3y Z 1 3x Y 2z 12 And X 2y 3z 5 Socratic



2




Algebra Expressions Year 9 Note 1 Expressions We



Math 4 Chapter 7 1 Solving Systems Of Linear Equations Oneclass




Factorise The Following 32 X Y Sq 2x 2y Mathematics Topperlearning Com W0twcmff




Implicit Differentiation Advanced Example Video Khan Academy




1 1 Yx3 3 2x Y 4 See How To Solve It At Qanda



Www Gocolumbia Edu Mrc Sec12 2 Pdf




Engineering Mathematics Notes




If 2 2x Y 16 And 2 X Y 32 Then Value Of My Is Brainly In
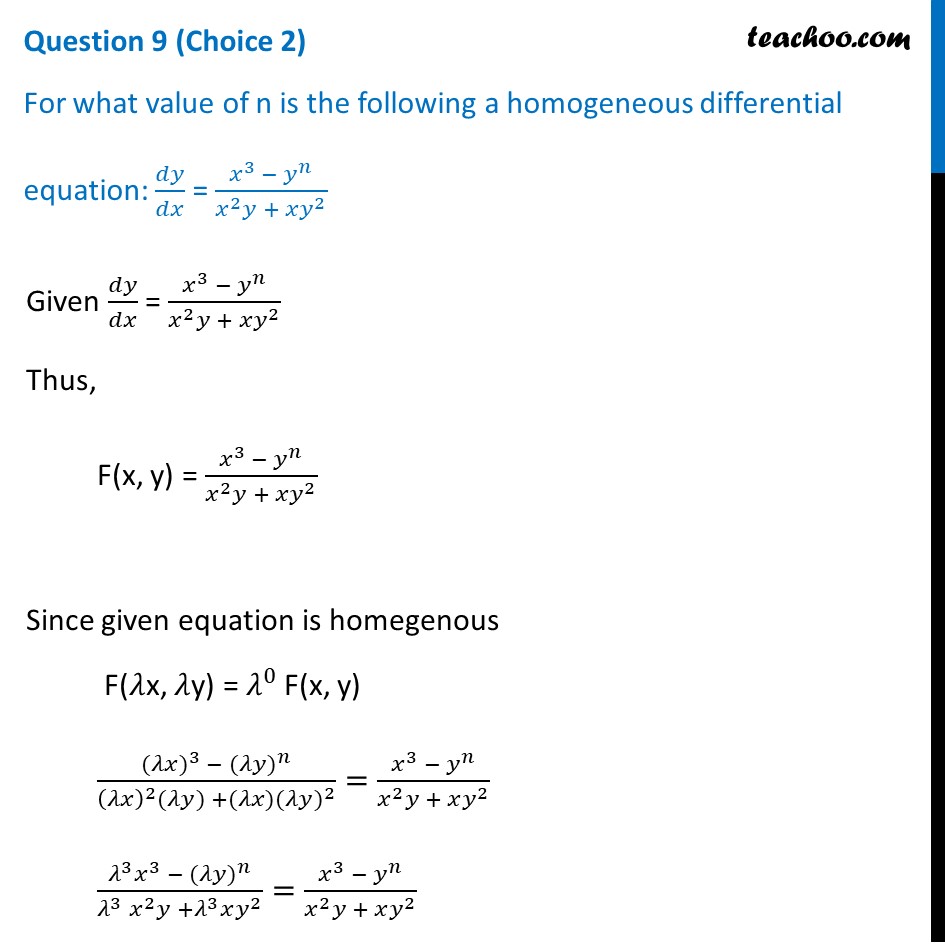



For What Value Of N Is Following A Homogeneous Differential Equation




100 Advanced Mathematics Ideas In 21 Advanced Mathematics Mathematics Advanced Physics




3 5 Solving Systems Of Equations In Three Variables Ppt Download



Www Thatonemathguy Com Uploads 7 0 1 3 Ch 3 Pdf




K Y 2 2x 2y



Solved Solve Each System Using Elimination 1 Xty 2 2 X 2y 3 3 2x Y 4 X Y 4 X Y 6 3x Y 2 4 X 2y 2 5 X 3y 3 6 X Course Hero
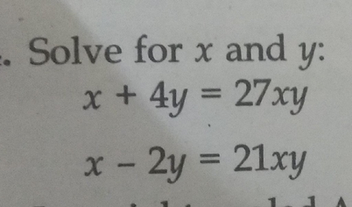



Solve For X And Y X 4y 27xy X 2y 21xy Scholr
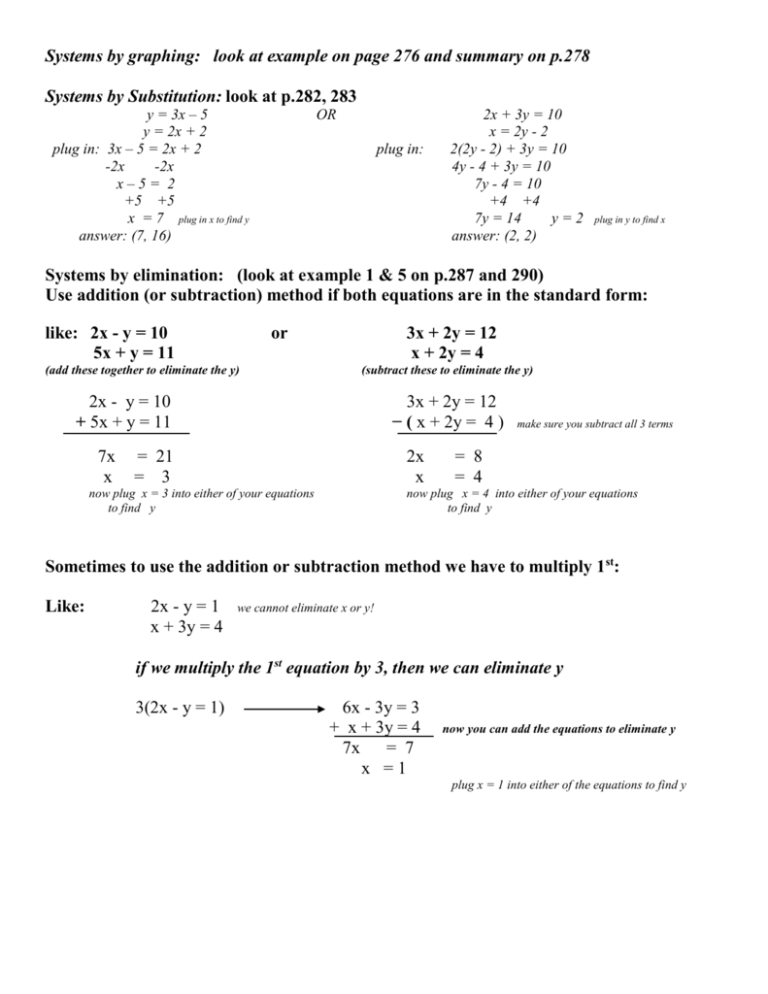



Notes For Systems




Answers




Graph Graph Inequalities With Step By Step Math Problem Solver



Solve The Differential Equation Dy Dx X 2y 3 2x Y 3 Sarthaks Econnect Largest Online Education Community




Answered Linear Equations 26 Chapter 1 Problems Bartleby



1




Solve Y 2 2x 2y Dx 2x 3 Xy Dy 0 Mathematics Stack Exchange




Beginarrayr 2x Y 3z 3 2x Y Z 5 X 2y 2z 22x 2z Gauthmath




Schaum S Outline College Algebra Pdf Document



2



Solve The Differential Equations Dy Dx X Y 3 2x 2y 5 Sarthaks Econnect Largest Online Education Community




Answered 0 Is Cnte Raph Costai Function 229en Bartleby



0 件のコメント:
コメントを投稿